An overview
Why multiple exposures?
- In certain environments where there are excessively bright or dark objects, a single exposure cannot guarantee optimal image quality for all objects. Therefore, additional exposures are necessary.
Principles of multiple exposures:
- The first exposure captures point clouds of some objects, and the second one captures point clouds of the others.
- It is recommended that under most circumstances, exposures of longer time precede those of shorter time to ensure more complete point clouds.
- Note that if the camera firmware version is higher than 2.0.2, it will prioritize exposures with longer time.
Steps in multiple exposures:
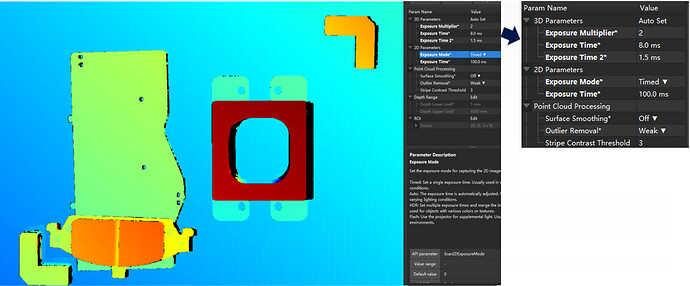
- Determine "Exposure Time” for bright objects.
- Determine “Exposure Time” for dull objects.
- Determine “Exposure Multiplier”, that is, the count of all exposures. Then set each “Exposure Time” in a way that creates an arithmetic progression for all values.
Disadvantages of multiple exposures:
- Overall imaging time is longer.
- Images’ quality is reliant on the first exposure after multiple exposures. If the first exposure results in noise, subsequent ones will not reduce such noise.
Principles of multiple exposures
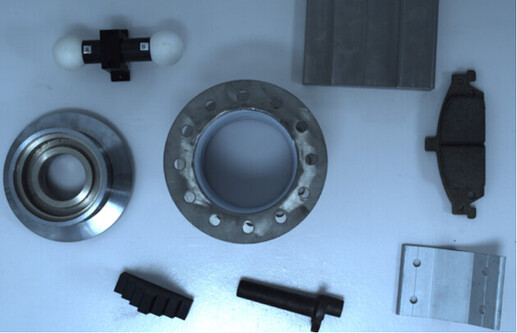
In environments where both dark and bright (reflective) objects coexist, there is often an incompatibility between the lighting conditions that suit each type of object. Therefore, one single exposure cannot accurately capture all objects in osuch an environment.
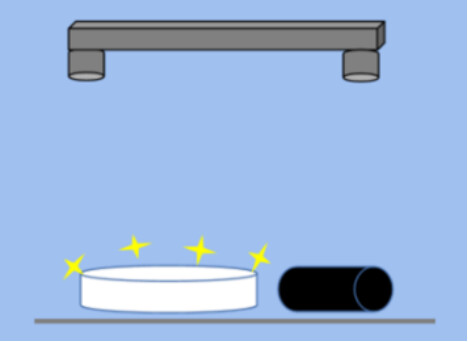
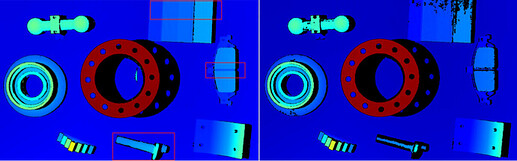
| Left image | Right image |
|---|---|
| “Exposure Multiplier”: 2 | “Exposure Multiplier”: 1 |
| “Exposure Time” 1: 6.0 ms | “Exposure Time”: 2.0 ms |
| “Exposure Time” 2: 2.0 ms | / |
Steps in multiple exposures:
- Find bright and dark objects on the scene.
- Determine appropriate “Exposure Time” for dull objects, for example, 8 ms.
- Determine appropriate “Exposure Time” for bright objects, for example, 1.5 ms.
- Set "Exposure Time” of the whole 3D object, for example, 8 + 1.5 ms.
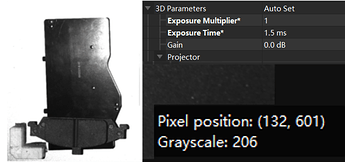
For dark objects, grayscale values depend on their flash images:
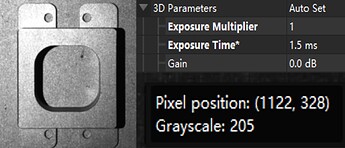
For bright objects, grayscale values depend on their flash images as well: